Bascal
Storyboard Text
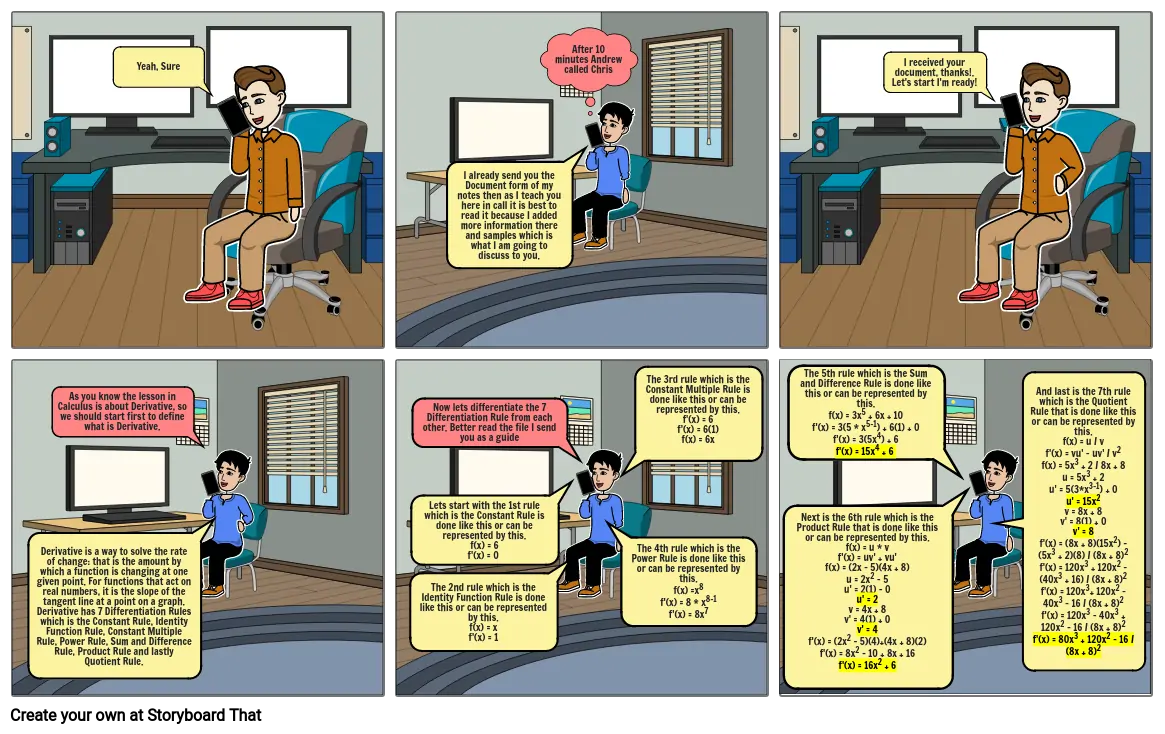
- Yeah, Sure
- I already send you the Document form of my notes then as I teach you here in call it is best to read it because I added more information there and samples which is what I am going to discuss to you.
- After 10 minutes Andrew called Chris
- I received your document, thanks!. Let's start I'm ready!
- Derivative is a way to solve the rate of change: that is the amount by which a function is changing at one given point. For functions that act on real numbers, it is the slope of the tangent line at a point on a graph. Derivative has 7 Differentiation Rules which is the Constant Rule, Identity Function Rule, Constant Multiple Rule, Power Rule, Sum and Difference Rule, Product Rule and lastly Quotient Rule.
- As you know the lesson in Calculus is about Derivative, so we should start first to define what is Derivative.
- The 2nd rule which is the Identity Function Rule is done like this or can be represented by this.f(x) = xf'(x) = 1
- Lets start with the 1st rule which is the Constant Rule is done like this or can be represented by this.f(x) = 6f'(x) = 0
- Now lets differentiate the 7 Differentiation Rule from each other. Better read the file I send you as a guide
- The 4th rule which is the Power Rule is done like this or can be represented by this.f(x) =x8f'(x) = 8 * x8-1f'(x) = 8x7
- The 3rd rule which is the Constant Multiple Rule is done like this or can be represented by this.f'(x) = 6f'(x) = 6(1)f(x) = 6x
- Next is the 6th rule which is the Product Rule that is done like this or can be represented by this.f(x) = u * vf'(x) = uv' + vu'f(x) = (2x - 5)(4x + 8)u = 2x2 - 5u' = 2(1) - 0u' = 2v = 4x + 8v' = 4(1) + 0v' = 4f'(x) = (2x2 - 5)(4)+(4x + 8)(2)f'(x) = 8x2 - 10 + 8x + 16f'(x) = 16x2 + 6
- The 5th rule which is the Sum and Difference Rule is done like this or can be represented by this.f(x) = 3x5 + 6x + 10f'(x) = 3(5 * x5-1) + 6(1) + 0f'(x) = 3(5x4) + 6f'(x) = 15x4 + 6
- And last is the 7th rule which is the Quotient Rule that is done like this or can be represented by this.f(x) = u / vf'(x) = vu' - uv' / v2f(x) = 5x3 + 2 / 8x + 8u = 5x3 + 2u' = 5(3*x3-1) + 0u' = 15x2v = 8x + 8v' = 8(1) + 0v' = 8 f'(x) = (8x + 8)(15x2) - (5x3 + 2)(8) / (8x + 8)2f'(x) = 120x3 + 120x2 - (40x3 + 16) / (8x + 8)2f'(x) = 120x3+ 120x2 - 40x3 - 16 / (8x + 8)2f'(x) = 120x3 - 40x3 + 120x2 - 16 / (8x + 8)2f'(x) = 80x3 + 120x2 - 16 / (8x + 8)2
Over 30 Million Storyboards Created

