Unknown Story
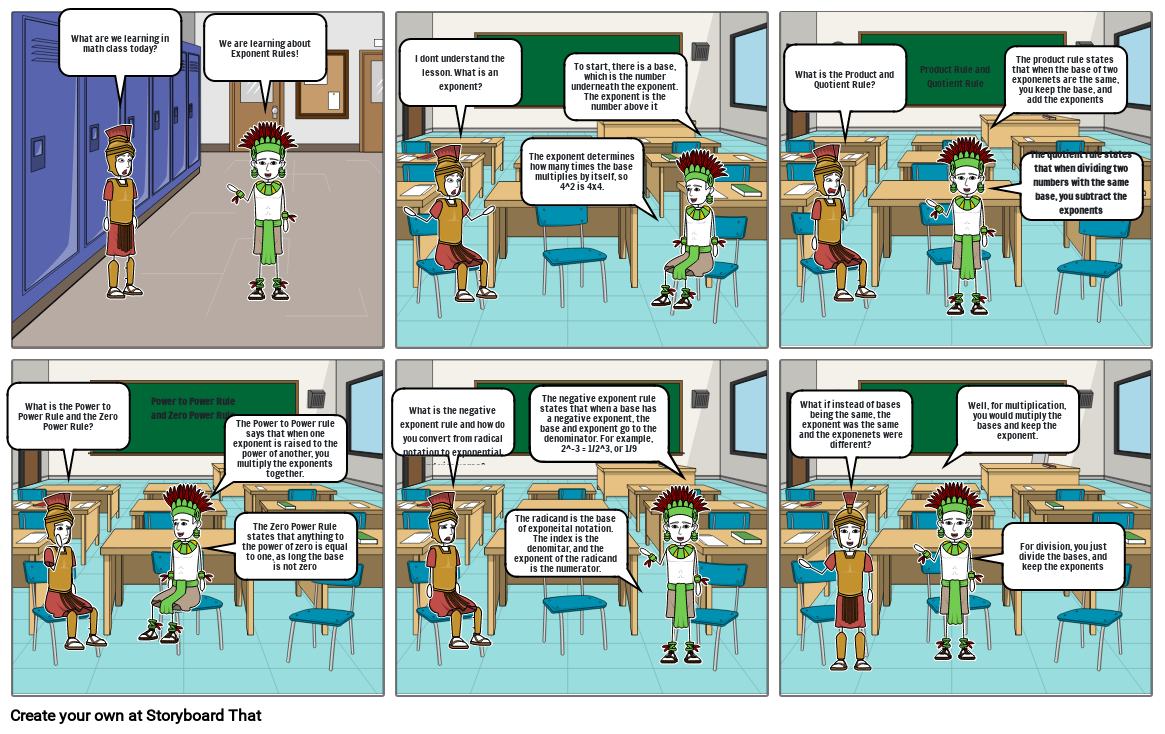
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
What are we learning in math class today?
We are learning about Exponent Rules!
To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
What is the Product and Quotient Rule?
The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
Power to Power Rule and Zero Power Rule
What is the Power to Power Rule and the Zero Power Rule?
The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
What if instead of bases being the same, the exponent was the same and the exponenets were different?
Well, for multiplication, you would mutiply the bases and keep the exponent.
For division, you just divide the bases, and keep the exponents
Storyboard Text
- What are we learning in math class today?
- We are learning about Exponent Rules!
- I dont understand the lesson. What is an exponent?
- The exponent determines how many times the base multiplies by itself, so 4^2 is 4x4.
- To start, there is a base, which is the number underneath the exponent. The exponent is the number above it
- What is the Product and Quotient Rule?
- Product Rule and Quotient Rule
- The quotient rule states that when dividing two numbers with the same base, you subtract the exponents
- The product rule states that when the base of two exponenets are the same, you keep the base, and add the exponents
- What is the Power to Power Rule and the Zero Power Rule?
- Power to Power Rule and Zero Power Rule
- The Zero Power Rule states that anything to the power of zero is equal to one, as long the base is not zero
- The Power to Power rule says that when one exponent is raised to the power of another, you multiply the exponents together.
- What is the negative exponent rule and how do you convert from radical notation to exponential and vice versa?
- The radicand is the base of exponeital notation. The index is the denomitar, and the exponent of the radicand is the numerator.
- The negative exponent rule states that when a base has a negative exponent, the base and exponent go to the denominator. For example, 2^-3 = 1/2^3, or 1/9
- What if instead of bases being the same, the exponent was the same and the exponenets were different?
- Well, for multiplication, you would mutiply the bases and keep the exponent.
- For division, you just divide the bases, and keep the exponents


