math
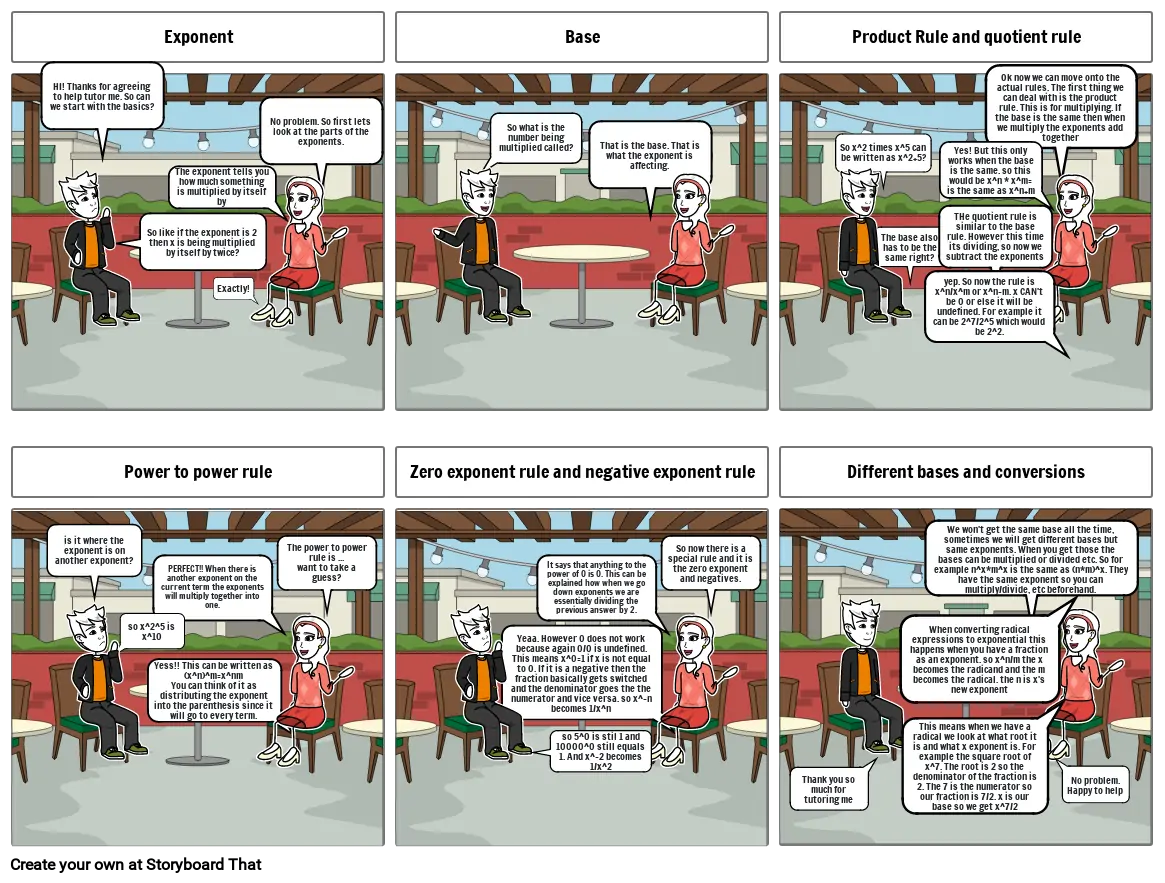
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Exponent
Base
Product Rule and quotient rule
Power to power rule
Zero exponent rule and negative exponent rule
Different bases and conversions
HI! Thanks for agreeing to help tutor me. So can we start with the basics?
No problem. So first lets look at the parts of the exponents.
So like if the exponent is 2 then x is being multiplied by itself by twice?
The exponent tells you how much something is multiplied by itself by
That is the base. That is what the exponent is affecting.
So x^2 times x^5 can be written as x^2+5?
So what is the number being multiplied called?
Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
The base also has to be the same right?
THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
The power to power rule is ...
want to take a guess?
PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
so x^2^5 is x^10
is it where the exponent is on another exponent?
Yess!! This can be written as (x^n)^m=x^nm
You can think of it as distributing the exponent into the parenthesis since it will go to every term.
Exactly!
yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
So now there is a special rule and it is the zero exponent and negatives.
It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
Thank you so much for tutoring me
No problem. Happy to help
Storyboard Text
- Exponent
- HI! Thanks for agreeing to help tutor me. So can we start with the basics?
- So like if the exponent is 2 then x is being multiplied by itself by twice?
- The exponent tells you how much something is multiplied by itself by
- Exactly!
- No problem. So first lets look at the parts of the exponents.
- Base
- So what is the number being multiplied called?
- That is the base. That is what the exponent is affecting.
- Product Rule and quotient rule
- So x^2 times x^5 can be written as x^2+5?
- The base also has to be the same right?
- yep. So now the rule is x^n/x^m or x^n-m. x CAN't be 0 or else it will be undefined. For example it can be 2^7/2^5 which would be 2^2.
- THe quotient rule is similar to the base rule. However this time its dividing, so now we subtract the exponents
- Yes! But this only works when the base is the same. so this would be x^n * x^m= is the same as x^n+m
- Ok now we can move onto the actual rules. The first thing we can deal with is the product rule. This is for multiplying. If the base is the same then when we multiply the exponents add together
- Power to power rule
- is it where the exponent is on another exponent?
- so x^2^5 is x^10
- Yess!! This can be written as (x^n)^m=x^nmYou can think of it as distributing the exponent into the parenthesis since it will go to every term.
- PERFECT!! When there is another exponent on the current term the exponents will multiply together into one.
- The power to power rule is ...want to take a guess?
- Zero exponent rule and negative exponent rule
- Yeaa. However 0 does not work because again 0/0 is undefined. This means x^0=1 if x is not equal to 0. If it is a negative then the fraction basically gets switched and the denominator goes the the numerator and vice versa. so x^-n becomes 1/x^n
- It says that anything to the power of 0 is 0. This can be explained how when we go down exponents we are essentially dividing the previous answer by 2.
- So now there is a special rule and it is the zero exponent and negatives.
- Different bases and conversions
- When converting radical expressions to exponential this happens when you have a fraction as an exponent. so x^n/m the x becomes the radicand and the m becomes the radical. the n is x's new exponent
- We won't get the same base all the time, sometimes we will get different bases but same exponents. When you get those the bases can be multiplied or divided etc. So for example n^x*m^x is the same as (n*m)^x. They have the same exponent so you can multiply/divide, etc beforehand.
- so 5^0 is stil 1 and 10000^0 still equals 1. And x^-2 becomes 1/x^2
- Thank you so much for tutoring me
- This means when we have a radical we look at what root it is and what x exponent is. For example the square root of x^7. The root is 2 so the denominator of the fraction is 2. The 7 is the numerator so our fraction is 7/2. x is our base so we get x^7/2
- No problem. Happy to help


