Complete The Square To Disarm
Storyboard Text
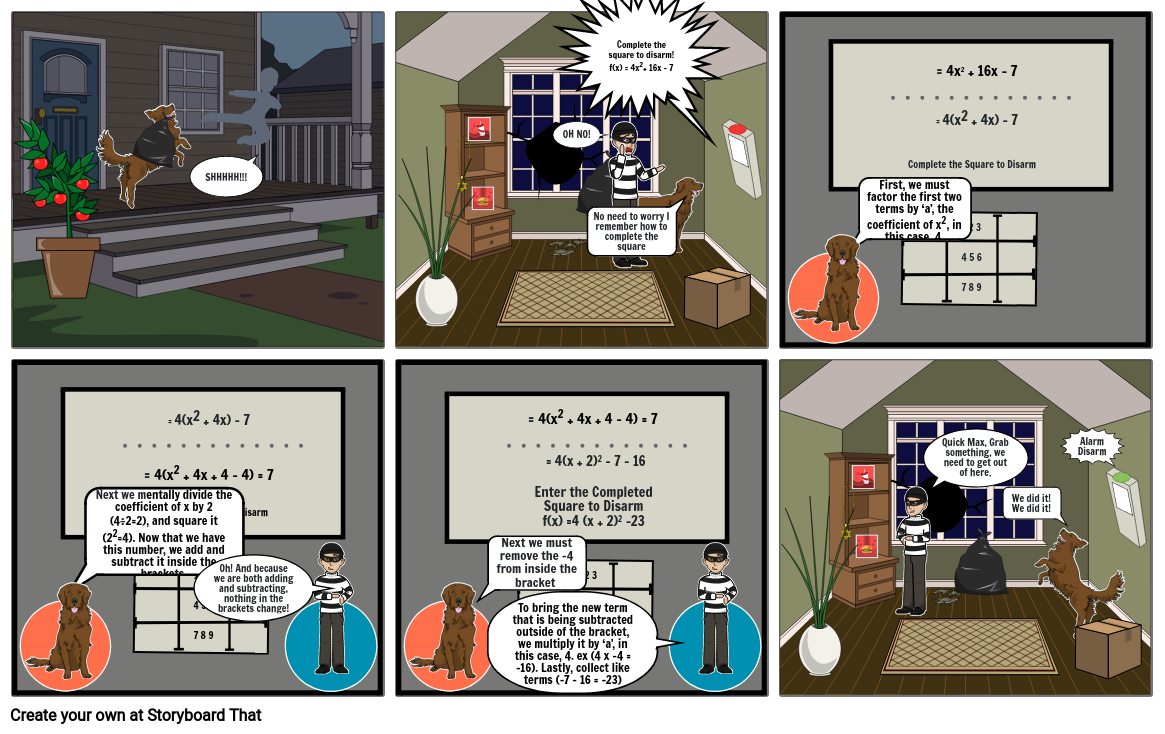
- SHHHHH!!!
- Complete the square to disarm!f(x) = 4x2 + 16x - 7
- No need to worry I remember how to complete the square
- OH NO!
- First, we must factor the first two terms by ‘a’, the coefficient of x2, in this case, 4.
- Complete the Square to Disarm
- = 4x2 + 16x - 7
- 1 2 34 5 67 8 9
- = 4(x2 + 4x) - 7
- Next we mentally divide the coefficient of x by 2 (4÷2=2), and square it (22=4). Now that we have this number, we add and subtract it inside the brackets.
- Complete the Square to Disarm
- = 4(x2 + 4x + 4 - 4) = 7
- 1 2 34 5 67 8 9
- = 4(x2 + 4x) - 7
- Oh! And because we are both adding and subtracting, nothing in the brackets change!
- Next we must remove the -4 from inside the bracket
- Enter the Completed Square to Disarm f(x) =4 (x + 2)2 -23
- = 4(x2 + 4x + 4 - 4) = 7
- = 4(x + 2)2 - 7 - 16
- 1 2 34 5 67 8 9
- To bring the new term that is being subtracted outside of the bracket, we multiply it by ‘a’, in this case, 4. ex (4 x -4 = -16). Lastly, collect like terms (-7 - 16 = -23)
- Quick Max, Grab something, we need to get out of here.
- We did it! We did it!
- Alarm Disarm
Over 30 Million Storyboards Created