Unknown Story
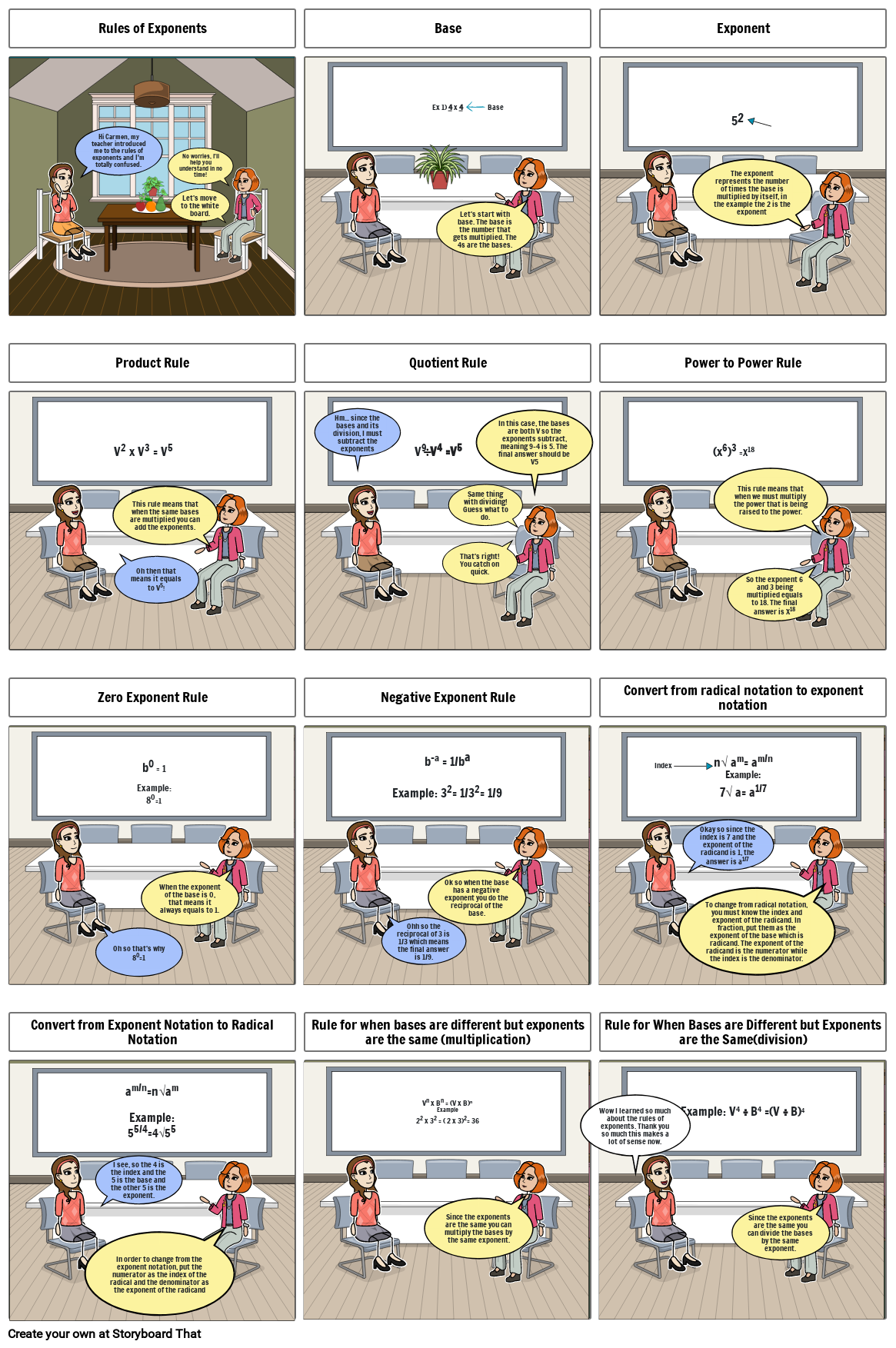
Storyboard Text
- Rules of Exponents
- Hi Carmen, my teacher introduced me to the rules of exponents and I'm totally confused.
- No worries, I'll help you understand in no time!
- Let's move to the white board.
- Base
- Ex 1) 4 x 4
- Let's start with base. The base is the number that gets multiplied. The 4s are the bases.
- Base
- Exponent
- The exponent represents the number of times the base is multiplied by itself, in the example the 2 is the exponent
- 52
- Product Rule
- V2 x V3 = V5
- This rule means that when the same bases are multiplied you can add the exponents.
- Quotient Rule
- Hm... since the bases and its division, I must subtract the exponents
- V9 V4 =V5
- Same thing with dividing! Guess what to do.
- In this case, the bases are both V so the exponents subtract, meaning 9-4 is 5. The final answer should be V5
- Power to Power Rule
- (x6)3 =X18
- This rule means that when we must multiply the power that is being raised to the power.
- Zero Exponent Rule
- b0 = 1Example: 80=1
- Oh then that means it equals to V5!
- Negative Exponent Rule
- b-a = 1/baExample: 32= 1/32= 1/9
- That's right! You catch on quick.
- Convert from radical notation to exponent notation
- Index
- n√ am= am/nExample: 7√ a= a1/7
- So the exponent 6 and 3 being multiplied equals to 18. The final answer is X18
- Convert from Exponent Notation to Radical Notation
- Oh so that's why 80=1
- When the exponent of the base is 0, that means it always equals to 1.
- Rule for when bases are different but exponents are the same (multiplication)
- Ohh so the reciprocal of 3 is 1/3 which means the final answer is 1/9.
- Ok so when the base has a negative exponent you do the reciprocal of the base.
- Rule for When Bases are Different but Exponents are the Same(division)
- To change from radical notation, you must know the index and exponent of the radicand. In fraction, put them as the exponent of the base which is radicand. The exponent of the radicand is the numerator while the index is the denominator.
- Okay so since the index is 7 and the exponent of the radicand is 1, the answer is a1/7
- am/n=n√amExample: 55/4=4√55
- In order to change from the exponent notation, put the numerator as the index of the radical and the denominator as the exponent of the radicand
- I see, so the 4 is the index and the 5 is the base and the other 5 is the exponent.
- Vn x Bn = (V x B)nExample 22 x 32 = ( 2 x 3)2= 36
- Since the exponents are the same you can multiply the bases by the same exponent.
- Wow I learned so much about the rules of exponents. Thank you so much this makes a lot of sense now.
- Example: V4 ÷ B4 =(V ÷ B)4
- Since the exponents are the same you can divide the bases by the same exponent.
Over 30 Million Storyboards Created